How Big Is An Electron

In the year 1908, while the great minds of natural philosophy were puzzling over how to understand the structure of atoms in terms of the recently-discovered electron, Vladimir Lenin (aye,that Vladimir Lenin) declared that:

The electron is as inexhaustible as the atom

Lenin was making a philosophical point, simply yous tin can view his declaration as something like a scientific prediction. Just as scientists of his era were discovering that atoms were made from smaller constituents like electrons, so likewise, he said, would nosotros somewhen find that the electron was fabricated from even smaller components. And on the cycle would go, with each new piece of matter being an "inexhaustible" source of new discovery.

It was a pretty reasonable expectation at the time, given the relentless progress of reductionist science. But so far, despite more than a century of work by scientists (including many presumably extra-motivated Soviets), nosotros still have no indication that the electron is made from anything else, or that information technology has any internal construction. Students of physics in the 21st century are taught that the electron is a point in space with certain properties — mass, charge, and spin — but that information technology cannot exist thought of as a spinning sphere or anything else that has a size and shape. The electron looks pretty exhausted.

In this post, though, I want to take Lenin's side, and ask the heretical question: "If the electron really is a real, concrete object with a finite size, then how large is information technology?" Non surprisingly, there is no clear reply to this question, only some of the candidate answers turn out to be pretty interesting.

Selection #1: The Bohr radius

If you're a physicist, and someone asks yous "how big is an electron?", and then the most canonically correct thing to say is "There is no concept of an electron size other than the spatial extent of the electron wavefunction. The size of the electron wavefunction is the electron size."

This opinion essentially amounts to telling someone that they need to stop trying to retrieve about a breakthrough electron as a physical object that you lot could hold in your hand like a baseball if only it was enlarged $10^{10}$ times. People who profess this stance are presumably too the ones who become annoyed by the popular declaration that "matter is 99.999% empty infinite."

So, if such a person were pressed to give a numerical value for the "size of the electron", they might say something like "Well, most electrons in the universe are bound into atoms. Then the typical 'size' of an electron is nigh the same as the typical size of an atom."

As has been discussed hither earlier, the typical size of an atom is given by the Bohr radius:

$a_B \sim 4 \pi \epsilon_0 \hbar^2/me^2$ .

[Here, $\hbar$ is Planck's constant, $\epsilon_0$ is the vacuum permittivity, and $m$ is the electron mass, and $e$ is the electron charge.]

Merely to remind you lot how this length calibration appears, you can figure out the rough size of an atom past remembering that an electron bound to an atom exists in a balance between two competing energies. Starting time, there is the bonny potential energy $U \sim -e^2/4 \pi \epsilon_0 r$ that pulls the electron toward the nucleus, and that gets stronger every bit the electron size $r$ (which is about the aforementioned as the average altitude between the electron and the nucleus) gets smaller. Second, in that location is the kinetic energy of the electron $KE \sim p^2/2m$ . The typical momentum of the electron gets larger as $r$ gets smaller, equally dictated by the Heisenberg uncertainty principle, $p \sim \hbar/r$ , which ways that the electron kinetic energy getslarger equally the atom size $r$ shrinks: $KE \sim \hbar^2/mr^2$ .

In the balanced state that is an atom, $PE$ and $KE$ are nigh the aforementioned, which ways that $e^2/4 \pi \epsilon_0 r \sim \hbar^2/mr^2$ . Solve for $r$ , and you lot get that the electron size is about equal to the Bohr radius, which numerically works out to near 1 Angstrom, or $10^{-10}$ m.

atom

So now we have our kickoff candidate answer to the question "how big is an electron?". If someone asks you this question, then you can sort of roll your eyes and so say "commonly, nearly $10^{-10}$ meters".

But let's keep going, and come across if at that place are any other concepts of an "electron size". Perhaps you don't really like the answer that "the size of the electron wavefunctionisthe electron size", because the electron wavefunction can be different in different situations, which means that this definition of "size" isn't really an immutable holding of all electrons. Maybe you lot prefer to call back about the electron as a tiny little ball, and the wavefunction as a sort of probability distribution that describes where the ball tends to be from time to time. If you insist on this point of view, then what tin can you say about the size of the ball?

Option #2: The classical electron radius

If yous're going to think about the electron as a tiny charged ball, and then there is one thing that should bother y'all: that brawl will take alot of energy.

To see why this is true, imagine the hypothetical process of assembling your tiny charged ball from a agglomeration of smaller pieces, each with a fraction of the total charge. Since the pieces have an electric repulsion from each other, and since you are bringing them very close to each other, the ball will be very hard to put together.

sphere_puzzle

In fact, the free energy required to "build" the electron is $E \sim e^2/4 \pi \epsilon_0 r$ , where $r$ is the electron size. And so the smaller the electron is, the harder it is to build, and the more energy gets stored in the form of electric repulsion between all the pieces. The big self-energy of the electron besides means that if the electron were to become "broken", then all the tiny pieces would fly apart from each other, and release a tremendous amount of energy.

But, in fact, by the end of the first decade of the 20th century, people already knew that a single electron stored a lot of energy. Einstein'due south famous equation, $E = mc^2$ , suggested that even a very small slice of matter was really an intensely full-bodied form of energy. I electron represents about $10^{-13}$ Joules (500,000 electron volts), which means that it only takes a few cups' worth of electrons to have enough rest mass free energy to equal the free energy of an atomic bomb. (Of course, if you always actually removed all the electrons from a few cups' worth of matter, you would create something much worse than an diminutive flop.)

So one of the early on attempts to estimate a size for the electron was to equate these two ideas. Perchance, the logic goes, the large "mass energy" of an electron is actually the same as the energy stored in the electrical repulsion of its constituent pieces. Equating those two expressions for the energy, $E = e^2/4\pi \epsilon_0 r = mc^2$ gives an estimate for $r$ that is called the "classical radius of the electron":

$r_c = e^2/(4 \pi \epsilon_0 m c^2) \sim 10^{-15}$ m

So that's our outset gauge for the intrinsic electron size: virtually $10^{-15}$ m.

This classical radius, by the way, works out to be well-nigh the same size as the typical size of an atomic nucleus. And this is, perchance, where we get the typical heart school picture show of the atom: if you lot call back about the electron using its classical radius, and so the moving picture y'all get is of a tiny negatively-charged speck orbiting some other equally tiny positively-charged speck, with most a cistron $10^5$ difference betwixt the speck sizes and the orbit sizes.

Choice #3: The Compton Wavelength

This picture of the electron as a little charged ball with size $10^{-15}$ meters seemed relatively okay upwardly until the 1920s, when it was discovered that the electron had more backdrop than just its charge and its mass. The electron also has what we now phone call a "spin".

Basically, the concept of spin comes downward to the fact that the electron is magnetic: it has a north pole and a south pole, and it creates a magnetic field around itself that is as large equally nigh 1 Tesla at a distance of 1 Angstrom abroad, and that decays in force as $1/(\text{distance})^3$ . Some other way of saying this aforementioned thing is that the electron has a "magnetic moment" whose value is equal to the "Bohr magneton", $\mu_B = e \hbar/2m$ . (Equally it turns out, this magnetic moment is substantially the same as the magnetic moment created by the orbit of an electron around a nucleus.)

At outset sight, this magnetic-ness of the electron doesn't seem like a problem. A charged sphere can create a magnetic field around itself, equally long as the sphere is spinning. Remember, for instance, that electrical currents create magnetic fields:

and that current is just moving electric accuse. So a spinning charged sphere also creates magnetic fields by virtue of the movement of its accuse-containing surface:

This sort of image is, in fact, why the electron's magnetic-ness was kickoff called "spin". People imagined that the piffling electron was actually spinning.

A problem arises with this picture pretty quickly, though. Namely, if the electron is very small, so in order for it to create a noticeable magnetic field it has to be spinningreally quickly. In item, the magnetic moment of a spinning sphere with charge $e$ is something like $\mu \sim e \omega r^2$ , where $\omega$ is the sphere'due south rotation frequency. If the sphere is spinning very quickly, and then $\omega$ is large, and the equator of the sphere is moving at a very fast speed $v \sim \omega r$ .

We know, however, that nothing can move faster than the speed of low-cal (including, presumably, the waistline of an electron). This puts a limit on how fast the electron tin can spin, which translates to a limit on how small the radius of the electron can be if we have any hope of explaining the observed electron magnetic field in terms of a physical rotation.

In detail, if y'all set $\mu = e \omega r^2 = e v r = \mu_B$ , and require that $v < c$ , then you get that the size of the electron $r$ must exist bigger than

$\lambda_c \sim \hbar/(m c) \approx 10^{-12}$ m.

In other words, if you hope to explain the electron magnetic field as coming from an actual spinning motion, then the size of the electron needs to be at least $10^{-12}$ yard. This is well-nigh a thousand times larger than the classical electron radius.

Coincidentally, this value $\lambda_c$ is chosen the "Compton wavelength", and information technology has another of import significant. The Compton wavelength is more or less the smallest distance to which y'all tin can confine an electron. If you endeavor to squeeze the electron into an even smaller distance, so its momentum will get so large (via the doubt principle) that information technology its kinetic energy volition exist larger than $mc^2$ . In this instance, there will be plenty energy to create (from the vacuum) a new electron-positron pair, and the newly-created positron tin but demolish the trapped electron while the newly-created electron flies away.

Option #4: the empiricist's view

At this point you may showtime to experience like none of the higher up estimates for the electron size seems meaningful. (Although don't be also harsh in discarding them: the concepts of the Bohr radius, the classical electron radius, and the Compton wavelength appear over and over over again in physics.)

So let's consider a purely empirical view: what practise experiments tell united states about how large an electron is?

I know of two types of experiments that qualify equally maxim something nigh the electron size. The first is a measurement of the electric dipole moment of the electron. The idea is that, perhaps, the electron does not e'er have its accuse bundled in a perfectly spherical way. Maybe its "shape" tin exist slightly disproportionate, like this:

asymmetric_electron

An asymmetric electron

In this case the electron would would want to align its "head" with an external electric field. The strength of the electron asymmetry is quantified by the electron dipole moment $d$ , which is defined as something like $d \sim ($ charge in the bottom one-half of the electron $-$ charge in the top half of the electron $) \times ($ electron size).

So far, nonetheless, experiments looking for a finite value of the electron dipole moment take non found one, and their experiments place an upper limit of $d < 10^{-30} \text{ electron charges} \times \text{meters}$ . This ways that either the electron is an extremely symmetric object (for example, a very perfect sphere) or its size is smaller than nearly $10^{-30}$ meters.

Some other set up of experiments looks for corrections to the way that the electron interacts with the vacuum. At a very conceptual level, an electron can absorb and re-emit photons from the vacuum, and this slightly alters its magnetic moment in a manner that is mind-bogglingly well-described. If the electron were to have a finite size, so this would alter its interaction with the vacuum a little bit, and the magnetic moment would very slightly change relative to our theories based on size-less electrons.

So far, however, experiments take seen no prove of such an effect. The accuracy of the experimental observations places an apparent upper limit on the electron size of about $10^{-18}$ m.

Selection #five: The Planck length

At this bespeak, seeing the apparent failure of the classical description to produce a coherent picture, and seeing the experimental advent of such spectacularly small numbers every bit $10^{-18}$ and $10^{-30}$ , you lot may be willing to carelessness Lenin's hope of an "inexhaustible" electron and only declare that the electron really is size-less. From now on, y'all may resolve, when you draw an electron, you'll draw information technology every bit a single pixel. Only only because you tin't draw information technology as a half-pixel.

Merely this brings upward the last, and strangest, question: what is the smallest conceivable length that anything tin can have? In other words, if the universe has a cardinal "pixel size", then what is it?

Of course, I don't know the respond to this question. Whether there really is a "smallest possible length" is interesting to consider, but at the moment it tin only be addressed with speculation. Nonetheless, we do know that at that place is a length scale below which our about basic theories of the universe finish making sense. This is called the "Planck length", $\ell_p$ . At distances smaller than the Planck length, nosotros are unable to draw even what empty infinite is like.

As I understand information technology, the Planck length trouble tin can be viewed like this. Our modern understanding of the vacuum (i.due east., of empty space) is that information technology contains one photon manner for every possible photon wavelength. This means that empty space is essentially total of photons of all conceivable energies and with all conceivable wavelengths.

Yet, photons with very small wavelength $\ell$ take very large free energy, $E \sim \hbar c/\ell$ . Information technology should therefore exist possible to convert that energy, if only for a cursory instant, into a large mass $M = E/c^2$ . (Afterward a curt time $t \sim \hbar/E$ , the mass will accept to disappear again and give its energy dorsum to the vacuum). If that mass is big enough, though, it can create a small blackness hole. A blackness hole gobbles upwardly all other photons around it if they have a wavelength smaller than the black hole's Schwarzschild radius, $R_s \sim G M/c^2$ . (Here, $G$ is Newton'south gravitational abiding). This starts to be a real trouble when $R_s$ gets as big as the wavelength of the photon that starting time created the black pigsty, because then the black pigsty can eat the original photon and all other photons with smaller wavelength.

Practise you lot see the problem with that (semi-contorted) logical sequence? If very short length scales be, then very loftier energy photons exist. But if high energy photons exist, so they should be able to create, for a brief moment, very high masses. Those loftier masses will create black holes. And those blackness holes will swallow upwardly all the high energy photons.

It's sort of a logical inconsistency.

As mentioned to a higher place, this problem outset arises when the Schwarzschild radius $R_s$ becomes equal to the wavelength $\ell$ of the photon that created information technology. If you work through the chain of algebra above, this will bring you lot to a length scale of

$\ell_p = \sqrt{\hbar G/c^3} \approx 10^{-35}$ m.

At any length calibration smaller than $\ell_p$ , we don't know what'southward going on. At such small length scales either quantum theory should be different from what nosotros know, or photons should exist unlike, or gravity should be different.

Or maybe at such pocket-sized length scales there is no good notion of continuous space at all. Such thinking, as I empathise information technology, gives ascension to lots of picturesque ideas about "quantum cream", and is the playground of (as still mostly non-existent) theories of quantum gravity.

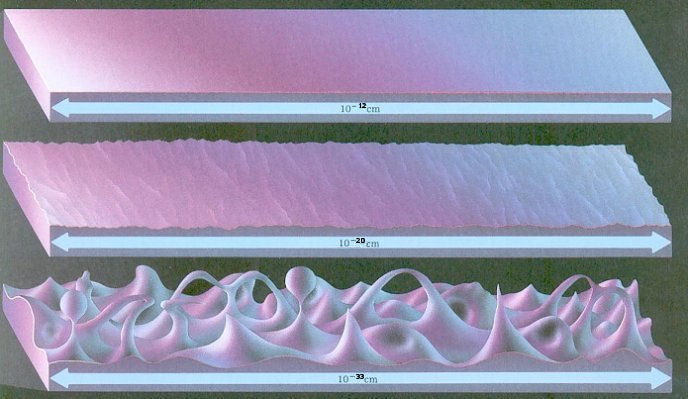
This picture is supposed to illustrate the idea that infinite might smooth and continuous until you try to look at it at the scale of the Planck length.

What shall we say about Lenin?

So in the finish, was Lenin right about the electron beingness "inexhaustible"? For the moment, information technology looks similar the reply is no, in the sense that in that location isn't really any serious candidate for the intrinsic size of the electron. In that sense, nosotros could all have saved some fourth dimension by just accepting the standard dogma that an electron is a sizeless betoken in space.

Merely I personally tend to resist dogmatism in all its forms, even the kind that is almost certainly correct. Because sometimes those heretical questions lead yous through all sorts of interesting ideas, ranging from from $10^{-10}$ meters down to $10^{-35}$ meters.

And if it becomes articulate some day that Lenin'southward argument really only works on the Planck scale, and so we tin probably say that his prediction came several centuries earlier its time.